ICU Physiology in 1000 Words: All Perfusion Is Local
Jon-Emile S. Kenny MD [@heart_lung]
About a year ago, I explored blood pressure – underscoring mean arterial pressure [MAP] as a determinant, but not necessarily an indicator, of perfusion. The inspiration for that post were these excellent reviews [1, 2] by the legendary Dr. Magder.
With the recent 65 Trial [3], a deeper exploration of tissue perfusion is warranted. Accordingly, this entry will provide a new way of visualizing the relationship between macro and micro hemodynamics – a conceptual approach which, hopefully, helps the clinician ponder therapeutic control of the circulation.
Independent & Dependent Variables
The first independent variable of the circulation is blood flow, or cardiac output; therefore, in figure 1A, cardiac output is plotted on the x-axis. In vivo, blood flow is stimulated by tissue oxygen demand [1, 4]. Therapeutically, cardiac output can be increased by either augmenting preload and/or using vasoactive medications.
The second independent variable of the circulation is tissue conductance. Conductance is the inverse of resistance; in other words, elevated tissue conductance is equivalent to decreased resistance [e.g. vasodilation] and vice versa. For simplicity, this analysis will consider a simplified two-tissue system each with variable conductance [i.e. tissue conductance is the slope of the blue and red lines in figure 1B]. In vivo, tissue conductance is changed by both systemic [e.g. adrenal release of catecholamines] and local [e.g. auto-regulation] factors. Therapeutically, tissue conductance may be mediated by the provision of vasoactives [e.g. norepinephrine, phenylephrine, etc.]. Importantly, tissue conductance is both highly dynamic and tissue-specific. Muscle, splanchnic tissue, the heart and kidney all have different baseline and capacities to adjust conductance [1, 5]; accordingly, each tissue may respond differentially in the face of identical changes in MAP.
The first dependent variable of the circulation is MAP – plotted on the y-axis in figure 1A. MAP is forged by blood flowing against ‘total vascular resistance’ [purple line, figure 1A]. For simplicity, this analysis will ignore the other components of the 3-element model of vascular impedance. Thus, the change in ‘total vascular resistance’ [purple line] is completely propelled by changes in tissue conductance. Consequently, ‘total vascular resistance’ [purple line] depicts a summed conductance of both tissue 1 [blue line, figure 1B] and tissue 2 [red line, figure 1B].
Crucially, MAP acts as an intermediary, independent variable for regional tissue flow – the second dependent variable of the circulation and plotted on the y-axis of figure 1B. Note that regional tissue flow is a function of the change in MAP and the tissue’s unique conductance. Figure 1 shows what happens where there is an increase in cardiac output [e.g. provision of intravenous fluids]. In 1A, cardiac output and, accordingly, MAP rises along the lumped vascular resistance curve. The increase in MAP – in figure 1B – then acts as an intermediary to increase regional tissue flow along each tissue’s individual conductance curve.
Figure 1: A. shows relationship between cardiac output and mean arterial pressure [macrovascular] while B. shows relationship between mean arterial pressure and regional tissue flow [microvascular]. SV is stroke volume, HR is heart rate. See text for details. The theoretical intercepts of the graphs are the mean systemic filling pressure [Pmsf] and closing pressure [Pc].
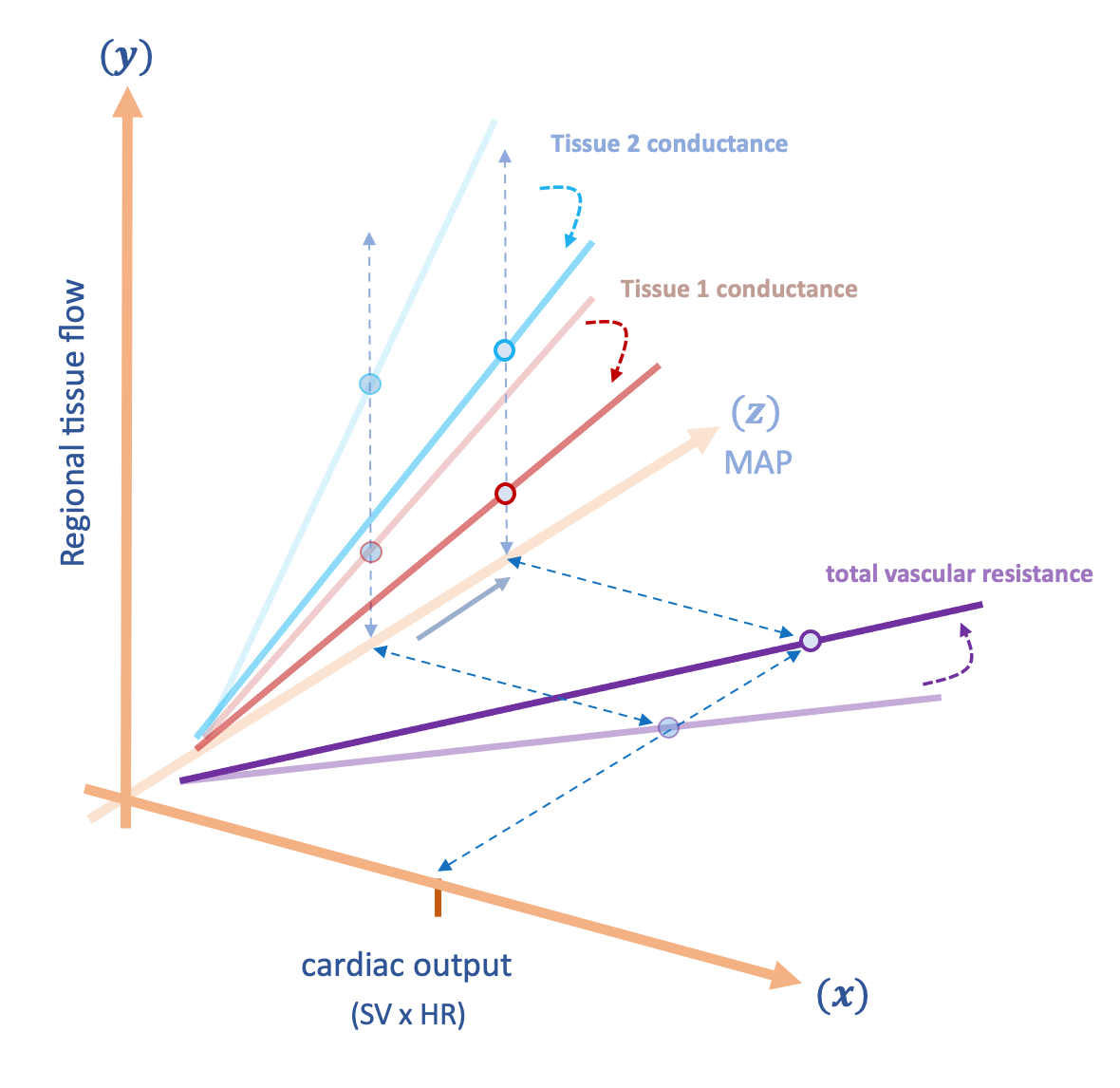
Macro-microvascular Dissociation
Figure 2 is identical to figure 1, however, figure 2 is combined into a single 3-dimensional plot. The x-z plane describes how cardiac output [x-axis] changes MAP [z-axis] which simultaneously acts upon the conductance of each individual tissue to drive local flow [y-axis]. Again, individual tissue conductance [i.e. slopes of blue and red lines, y-z plane] combine to determine the lumped ‘total vascular resistance’ [slope of purple line, x-z plane].
From this graphic, it should be clear that a change in macrovascular hemodynamics [i.e. cardiac output on x-axis] is expected to result in different microvascular hemodynamics [regional tissue flow on y-axis]. In other words, macro-microvascular dissociation is normal and expected; ‘coherence’ should not be assumed to exist [6]! Investigations trying to relate the two are almost certainly doomed to fail.
Figure 2: The same as figure 1, combined into 3D graphic. This shows the relationship between macrovascular hemodynamics on the x-axis and microvascular hemodynamics on the y-axis. Mean arterial pressure is the z-axis directed into the page. Faded lines and points represent baseline condition and emboldened lines and points represent response to intervention [here an increase in cardiac output].
Selective Vasoconstriction
Figure 3 shows the hypothetical effect of selectively vasoconstricting tissue 2 [i.e. decreased conductance of tissue 2]. The fall in tissue conductance on the y-z plane increases ‘total vascular resistance’ on the x-z plane. If cardiac output is unchanged, then MAP rises – increasing the regional flow of tissue 1 [red curve] while decreasing the flow to tissue 2 [blue curve]. An imperfect clinical correlation may be the provision of angiotensin II [7, 8] – selectively reducing the conductance of the kidney. Such physiology highlights the complexities of ‘tissue perfusion.’ A decrease in flow, in an organ like the kidney, may paradoxically improve glomerular perfusion when there is pathological shunting. By contrast, a selective fall in conductance of vascular beds supplying the fingers or the visceral organs could lead to digital and mesenteric ischemia, respectively.
Figure 3: A selective reduction in conductance [increased resistance] of tissue 2. Faded points and lines represent baseline while emboldened points and lines represent effect of intervention. MAP rises [z-axis], but only tissue 1 sees an increase in flow. See text for details. For simplicity, these graphs assume no change in Pmsf or Pc.
Global Vasoconstriction
Figure 4 illustrates global reduction in tissue conductance. A clinical example of this might be high dose phenylephrine [9] or methylene blue. Here, diminished tissue conductance greatly augments total vascular resistance on the x-z plane. If cardiac output is unchanged, then MAP [z-axis] rises significantly; however, despite a boosted MAP, regional tissue flow [y-axis] falls for both tissues. If cardiac output were to drop [e.g. from high afterload], regional tissue flow would diminish further [not pictured]. Note that norepinephrine could have a similar effect; cardiac output may rise [10] or fall [11] in response to norepinephrine.
Figure 4: Global vasoconstriction depicted as reduced conductance [increased resistance] of tissue 1 and 2. Faded points and lines represent baseline while emboldened points and lines represent effect of intervention. MAP rises considerably [z-axis], but regional tissue flow [y-axis] falls for both tissues. See text for details.
Global Vasodilation
On the other hand, if all tissues were to increase their conductance in exact proportion, the fall in MAP [z-axis] is offset and local tissue flow [y-axis] can be preserved or even improved [see figure 5]. This physiology may be how some individuals can live with unusually low mean arterial pressures [1, 2]. Thus, the key insult to tissues secondary to vasodilation is when some vascular beds increase their conductance relative to others, for the latter will have their local flow impaired the most, especially if cardiac output cannot increase to compensate. This is most prominent for tissues with a high baseline conductance [e.g. kidneys].
Figure 5: Global vasodilation - both tissues increase their conductances in proportion. Faded points and lines represent baseline while emboldened points and lines represent effect of intervention. Had only tissue 1 increased its conductance, the fall in MAP [z-axis] would have decreased tissue 2's regional flow; because both tissues increase conductance, the fall in MAP is met by increased flow for both tissues [y-axis]. See text for details.
Conclusion
In summary, MAP is determined by lumped vascular resistance while simultaneously driving local tissue perfusion based on regional tissue conductance. Decreased tissue conductance can raise MAP yet diminish local flow, while increased tissue conductance may lower MAP with preserved local flow. For these reasons, resuscitation based on markers of regional perfusion [e.g. capillary refill] and the concept of ‘permissive hypotension [12]’ carry some physiological weight.
We see then, as we enter another U.S. Presidential election, that we might think about hemodynamics as the late Tip O’Neill famously described running for office: all perfusion, like politics, is local.
Please see other posts in this series,
JE
Dr. Kenny is the cofounder and Chief Medical Officer of Flosonics Medical; he also the creator and author of a free hemodynamic curriculum at heart-lung.org
References
Magder SA: The highs and lows of blood pressure: toward meaningful clinical targets in patients with shock. Critical care medicine 2014, 42(5):1241-1251.
Magder S: The meaning of blood pressure. Critical Care 2018, 22(1):257.
Lamontagne F, Richards-Belle A, Thomas K et al: Effect of Reduced Exposure to Vasopressors on 90-Day Mortality in Older Critically Ill Patients With Vasodilatory Hypotension: A Randomized Clinical Trial. JAMA 2020.
Magder SA: The ups and downs of heart rate. Critical care medicine 2012, 40(1):239-245.
Magder S: Pressure-flow relations of diaphragm and vital organs with nitroprusside-induced vasodilatation. Journal of Applied Physiology 1986, 61(2):409-416.
Bennett VA, Vidouris A, Cecconi M: Effects of Fluids on the Macro-and Microcirculations. Critical Care 2018, 22(1):74.
Khanna A, English SW, Wang XS et al: Angiotensin II for the treatment of vasodilatory shock. New England Journal of Medicine 2017, 377(5):419-430.
Corrêa TD, Takala J, Jakob SM: Angiotensin II in septic shock. Critical care 2015, 19(1):98.
Magder S: Phenylephrine and tangible bias. Anesthesia & Analgesia 2011, 113(2):211-213.
Persichini R, Silva S, Teboul J-L et al: Effects of norepinephrine on mean systemic pressure and venous return in human septic shock. Critical care medicine 2012, 40(12):3146-3153.
Guarracino F, Bertini P, Pinsky MR: Cardiovascular determinants of resuscitation from sepsis and septic shock. Critical Care 2019, 23(1):118.
Marshall JC: Choosing the Best Blood Pressure Target for Vasopressor Therapy. JAMA 2020.